Understanding Current Electricity With Conductive Dough

I've noticed something about myself. Between August and October, I don't write much here.

I blame school.

If I had a regular reader of this blog, I would apologize profusely. But since this space likes to pretend it's part of the deep web, even though it isn't, I'm not sorry at all.

I blame this blog.

You hear that blog? I'm not impressed with your superpower choice.

When choosing between the superpowers of invisibility or the power of flight, this blog has chosen invisibility

Onto the program!

After practicing extensively with batteries, lights, wires, and circuits in general, last week my kids made a batch of conductive dough. Why? Well, making food-like products is a great math unit in measurement. Also the dough replaces wires in a traditional circuit, and it's a challenge to extrapolate what the kids learned with current electricity and wires and apply it to another substance. 

How do you make conductive dough? From the squishy circuits website, mix together:

1 cup of water,

1 and a half cups of flour,

1/4 cup of salt,

3 Tablespoons of Cream of Tartar or 9 Tablespoons of Lemon Juice,

1 Tablespoon of Vegetable Oil,

And maybe some food coloring

I gave all the groups a tablespoon, and asked them to choose and rationalize their second measuring tool; either a cup, a 1/2 cup, or a 1/4 cup.

Most groups chose the 1/4 cup. Yay!

For an extra challenge, I asked a couple groups to make two servings in one batch.

I'm dealing with a small sample size, but it seems like every year two things will happen:

1) One group will totally not follow the recipe, and

2) Another group will spill their contents on our carpeted floor. 

Both problems are salvageable. The former can be corrected by adding extra water and burning it off while it bakes, and the latter problem can be remedied by scooping it back in the bowl. 

The end result... before it was cooked, and after it was scooped back in the bowl

But before we try to figure out how conductive dough works, I give them this photo problem:
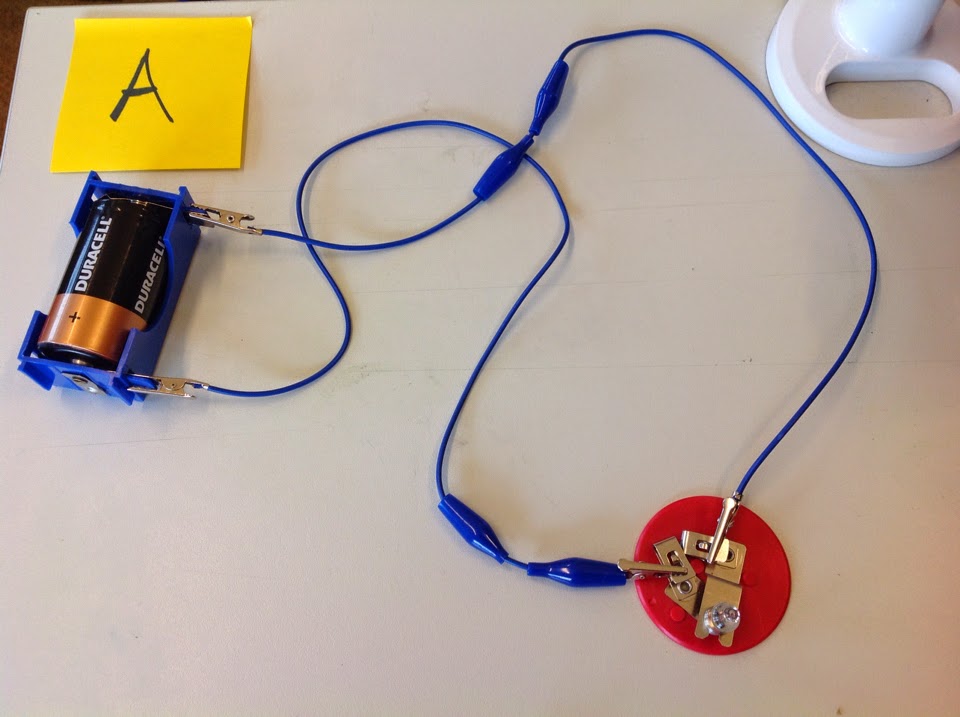
In the picture, all components are working. The batteries, bulb, wires, and holders all work great. But the light isn't on. Given what you know about electricity and how it flows in a circuit, why doesn't this circuit work?

The crossed wires might be confusing, but that's a red herring

I don't really expect many to figure out what's wrong even with an extensive knowledge of the tools here and a passing knowledge of current electricity.

The reason is because of that red bulb holder. The two pieces of metal are touching, and electricity will always follow the path of least resistance. It's much easier for current electricity to go through a conductor such as metal than a resistor like the bulb. So it takes the easy path. The bulb has no current passing through it to light and heat the filament inside. 

It's a point I'd like the children to internalize. To check if they do, next I give them a glob of conductive dough, a battery pack, and an LED. I ask them to think about this picture, the problem, and the solution, and to extrapolate that knowledge to make a squishy circuit.

They planned it out, made a diagram, drew the path of electricity to the squishy circuit, and most came up with is some variation of this:

"Ryan, I think the bulb is broken"

So I brought them back to the first photo, emphasizing the path of electricity. Then asked them reconsider their squishy circuit. Most then were able to see the problem in a different way, and their trial and error had a lot more purpose. Eventually they reached a conclusion: You have to force the current into the bulb, and sticking it into the conductive dough just won't work.

What does work? This:

"Huzzah!"

I love this lesson. I love not only playing with other materials out of the students' comfort zone, but extrapolating lessons from one set of materials and applying it to another set. If only there was some sort of non-messy liquid conductor that could pose the same kind of challenge through extrapolation...

oh, wait.